

I remember reading it and thinking how much easier Barrett O’Neill’s differential geometry book would have been if I’d mastered Baxandall and Liebeck first. This is the kind of book you wish you’d had when you learned vector calculus.
And it’s all topped off with lots of equally terrific exercises, none too hard. Even better, the presentation is extremely example driven-there are literally hundreds of examples throughout-from both mathematics and classical physics. The language of linear algebra is used freely and without reservation, careful definitions are given and the presentation is still extremely visual as each concept is given with several graphs. The standard concepts-such as chain rule, the inverse and implicit function theorems and multiple integrals-are presented several times at different levels of generality.
#Vector calculus full
This way the presentation begins in the simplest manner and gradually achieves full generality. The book has a “spiral ascent” structure-it begins with the simplest kinds of functions of several variables, namely the real valued maps of $R^2$ and proceeds through vector valued maps in $R^2$ then maps from $R^2$ to $R^3$, etc., culminating with a careful study of vector valued functions, derivatives and differentials, and line and surface integrals in general $R^n$. This makes a world of difference as it allows them to present the elements of several variable calculus as the study of certain linear transformations (the general derivative, the differential) between subspaces of $R^n$. The authors presume the students have strong backgrounds in linear algebra and a careful study of calculus using $\epsilon-\delta$ limit definitions. In any event, this wonderful textbook is rooted in the UK university system and therefore begins at a higher level them most American texts. Like I said above, this is a highly questionable assumption to say the least and even if it were true, a careful study of vector valued functions in R2 and R3 first might allow students to transition directly to modern differential topology and geometry without the “forms for dummies” approach. Such books are obviously mostly written for engineering and non-mathematics students, assuming that mathematics and serious physics majors would opt into courses and texts based in differentiable manifolds. Most of them are afraid to discuss linear transformations and vector spaces, for God’s sake. The overwhelming majority of such books are basically plug and chug books that may as well have been written in the 19th century and avoid any hard theory like Lyme Disease.

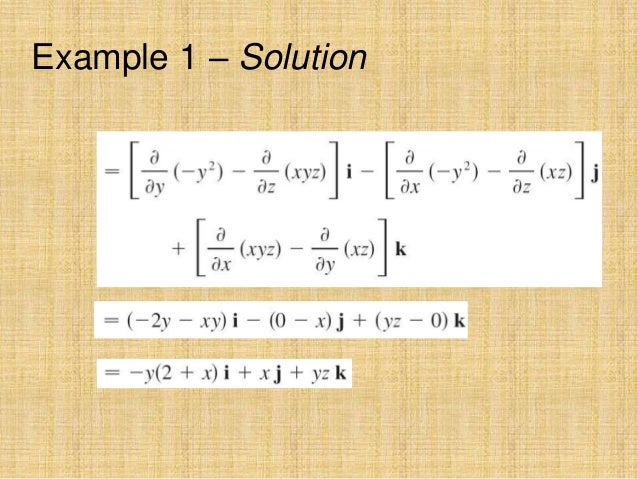
I stumbled across this terrific and very underrated book while searching for a modern treatment of functions of several variables that could be used by bright undergraduates without the use of manifolds or differential forms. The best introductory textbook on multivariable calculus for the rank beginner that I know is Vector Calculus by Peter Baxandall and Hans Liebeck. Div F = ∇ ⋅ F = ( ∂ ∂ x, ∂ ∂ y, ∂ ∂ z ) ⋅ ( F x, F y, F z ) = ∂ F x ∂ x + ∂ F y ∂ y + ∂ F z ∂ z.


 0 kommentar(er)
0 kommentar(er)
